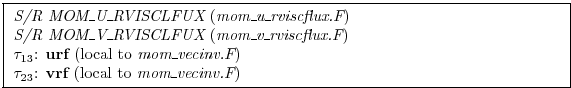Home Contact Us Site Map  
 
       
    next up previous contents
Next: 2.16 Tracer equations Up: 2.15 Vector invariant momentum Previous: 2.15.7 Horizontal dissipation   Contents

2.15.8 Vertical dissipation

Currently, this is exactly the same code as the flux form equations.

$\displaystyle G_u^{v-diss}$ $\displaystyle =$ $\displaystyle \frac{1}{\Delta r_f h_w} \delta_k \tau_{13}$ (2.162)
$\displaystyle G_v^{v-diss}$ $\displaystyle =$ $\displaystyle \frac{1}{\Delta r_f h_s} \delta_k \tau_{23}$ (2.163)

represents the general discrete form of the vertical dissipation terms.

In the interior the vertical stresses are discretized:

$\displaystyle \tau_{13}$ $\displaystyle =$ $\displaystyle A_v \frac{1}{\Delta r_c} \delta_k u$ (2.164)
$\displaystyle \tau_{23}$ $\displaystyle =$ $\displaystyle A_v \frac{1}{\Delta r_c} \delta_k v$ (2.165)

\fbox{ \begin{minipage}{4.75in} {\em S/R MOM\_U\_RVISCLFUX} ({\em mom\_u\_rviscf... ....F}) \par $\tau_{23}$: {\bf vrf} (local to {\em mom\_vecinv.F}) \end{minipage} }


mitgcm-support@mitgcm.org
Copyright © 2006 Massachusetts Institute of Technology Last update 2011-01-09