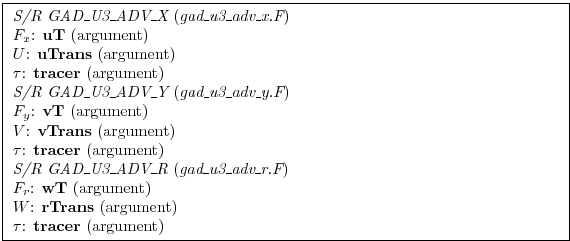Home Contact Us Site Map  
 
       
    next up previous contents
Next: 2.17.3 Centered fourth order Up: 2.17 Linear advection schemes Previous: 2.17.1 Centered second order   Contents

2.17.2 Third order upwind bias advection

Upwind biased third order advection offers a relatively good compromise between accuracy and smoothness. It is not a ``positive'' scheme meaning false extrema are permitted but the amplitude of such are significantly reduced over the centered second order method.

The third order upwind fluxes are discretized:

$\displaystyle F_x$ $\displaystyle =$ $\displaystyle U \overline{\tau - \frac{1}{6} \delta_{ii} \tau}^i + \frac{1}{2} \vert U\vert \delta_i \frac{1}{6} \delta_{ii} \tau$ (2.178)
$\displaystyle F_y$ $\displaystyle =$ $\displaystyle V \overline{\tau - \frac{1}{6} \delta_{ii} \tau}^j + \frac{1}{2} \vert V\vert \delta_j \frac{1}{6} \delta_{jj} \tau$ (2.179)
$\displaystyle F_r$ $\displaystyle =$ $\displaystyle W \overline{\tau - \frac{1}{6} \delta_{ii} \tau}^k + \frac{1}{2} \vert W\vert \delta_k \frac{1}{6} \delta_{kk} \tau$ (2.180)

At boundaries, $ \delta_{\hat{n}} \tau$ is set to zero allowing $ \delta_{nn}$ to be evaluated. We are currently examine the accuracy of this boundary condition and the effect on the solution.

\fbox{ \begin{minipage}{4.75in} {\em S/R GAD\_U3\_ADV\_X} ({\em gad\_u3\_adv\_x.... ...bf rTrans} (argument) \par $\tau$: {\bf tracer} (argument) \par \end{minipage} }


mitgcm-support@mitgcm.org
Copyright © 2006 Massachusetts Institute of Technology Last update 2011-01-09