Home Contact Us Site Map  
 
       
    next up previous contents
Next: 2.10 Variants on the Up: 2. Discretization and Algorithm Previous: 2.8 Staggered baroclinic time-stepping   Contents


2.9 Non-hydrostatic formulation

The non-hydrostatic formulation re-introduces the full vertical momentum equation and requires the solution of a 3-D elliptic equations for non-hydrostatic pressure perturbation. We still intergrate vertically for the hydrostatic pressure and solve a 2-D elliptic equation for the surface pressure/elevation for this reduces the amount of work needed to solve for the non-hydrostatic pressure.

The momentum equations are discretized in time as follows:

$\displaystyle \frac{1}{\Delta t} u^{n+1} + g \partial_x \eta^{n+1} + \partial_x \phi_{nh}^{n+1}$ $\displaystyle =$ $\displaystyle \frac{1}{\Delta t} u^{n} + G_u^{(n+1/2)}$ (2.53)
$\displaystyle \frac{1}{\Delta t} v^{n+1} + g \partial_y \eta^{n+1} + \partial_y \phi_{nh}^{n+1}$ $\displaystyle =$ $\displaystyle \frac{1}{\Delta t} v^{n} + G_v^{(n+1/2)}$ (2.54)
$\displaystyle \frac{1}{\Delta t} w^{n+1} + \partial_r \phi_{nh}^{n+1}$ $\displaystyle =$ $\displaystyle \frac{1}{\Delta t} w^{n} + G_w^{(n+1/2)}$ (2.55)

which must satisfy the discrete-in-time depth integrated continuity, equation 2.16 and the local continuity equation

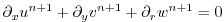$\displaystyle \partial_x u^{n+1} + \partial_y v^{n+1} + \partial_r w^{n+1} = 0$ (2.56)

As before, the explicit predictions for momentum are consolidated as:
$\displaystyle u^*$ $\displaystyle =$ $\displaystyle u^n + \Delta t G_u^{(n+1/2)}$  
$\displaystyle v^*$ $\displaystyle =$ $\displaystyle v^n + \Delta t G_v^{(n+1/2)}$  
$\displaystyle w^*$ $\displaystyle =$ $\displaystyle w^n + \Delta t G_w^{(n+1/2)}$  

but this time we introduce an intermediate step by splitting the tendancy of the flow as follows:
$\displaystyle u^{n+1} = u^{**} - \Delta t \partial_x \phi_{nh}^{n+1}$   $\displaystyle u^{**} = u^{*} - \Delta t g \partial_x \eta^{n+1}$ (2.57)
$\displaystyle v^{n+1} = v^{**} - \Delta t \partial_y \phi_{nh}^{n+1}$   $\displaystyle v^{**} = v^{*} - \Delta t g \partial_y \eta^{n+1}$ (2.58)

Substituting into the depth integrated continuity (equation 2.16) gives

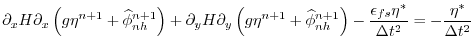$\displaystyle \partial_x H \partial_x \left( g \eta^{n+1} + \widehat{\phi}_{nh}... ... \right) - \frac{\epsilon_{fs}\eta^*}{\Delta t^2} = - \frac{\eta^*}{\Delta t^2}$ (2.59)

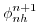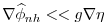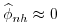which is approximated by equation 2.20 on the basis that i) $ \phi_{nh}^{n+1}$ is not yet known and ii) $ \nabla \widehat{\phi}_{nh} << g \nabla \eta$ . If 2.20 is solved accurately then the implication is that $ \widehat{\phi}_{nh} \approx 0$ so that thet non-hydrostatic pressure field does not drive barotropic motion.

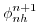The flow must satisfy non-divergence (equation 2.56) locally, as well as depth integrated, and this constraint is used to form a 3-D elliptic equations for $ \phi_{nh}^{n+1}$ :

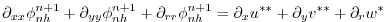$\displaystyle \partial_{xx} \phi_{nh}^{n+1} + \partial_{yy} \phi_{nh}^{n+1} + \... ...{rr} \phi_{nh}^{n+1} = \partial_x u^{**} + \partial_y v^{**} + \partial_r w^{*}$ (2.60)

The entire algorithm can be summarized as the sequential solution of the following equations:

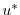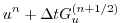$\displaystyle u^{*}$ $\displaystyle =$ $\displaystyle u^{n} + \Delta t G_u^{(n+1/2)}$ (2.61)
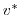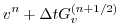$\displaystyle v^{*}$ $\displaystyle =$ $\displaystyle v^{n} + \Delta t G_v^{(n+1/2)}$ (2.62)
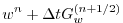$\displaystyle w^{*}$ $\displaystyle =$ $\displaystyle w^{n} + \Delta t G_w^{(n+1/2)}$ (2.63)
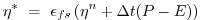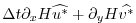$\displaystyle \eta^* ~ = ~ \epsilon_{fs} \left( \eta^{n} + \Delta t (P-E) \right)$ $\displaystyle -$ $\displaystyle \Delta t \partial_x H \widehat{u^{*}} + \partial_y H \widehat{v^{*}}$ (2.64)
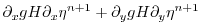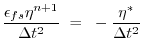$\displaystyle \partial_x g H \partial_x \eta^{n+1} + \partial_y g H \partial_y \eta^{n+1}$ $\displaystyle -$ $\displaystyle \frac{\epsilon_{fs} \eta^{n+1}}{\Delta t^2} ~ = ~ - \frac{\eta^*}{\Delta t^2}$ (2.65)
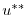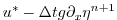$\displaystyle u^{**}$ $\displaystyle =$ $\displaystyle u^{*} - \Delta t g \partial_x \eta^{n+1}$ (2.66)
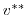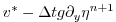$\displaystyle v^{**}$ $\displaystyle =$ $\displaystyle v^{*} - \Delta t g \partial_y \eta^{n+1}$ (2.67)
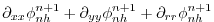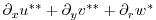$\displaystyle \partial_{xx} \phi_{nh}^{n+1} + \partial_{yy} \phi_{nh}^{n+1} + \partial_{rr} \phi_{nh}^{n+1}$ $\displaystyle =$ $\displaystyle \partial_x u^{**} + \partial_y v^{**} + \partial_r w^{*}$ (2.68)
$\displaystyle u^{n+1}$ $\displaystyle =$ $\displaystyle u^{**} - \Delta t \partial_x \phi_{nh}^{n+1}$ (2.69)
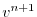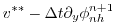$\displaystyle v^{n+1}$ $\displaystyle =$ $\displaystyle v^{**} - \Delta t \partial_y \phi_{nh}^{n+1}$ (2.70)
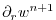$\displaystyle \partial_r w^{n+1}$ $\displaystyle =$ $\displaystyle - \partial_x u^{n+1} - \partial_y v^{n+1}$ (2.71)

where the last equation is solved by vertically integrating for $ w^{n+1}$ .

next up previous contents
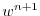Next: 2.10 Variants on the Up: 2. Discretization and Algorithm Previous: 2.8 Staggered baroclinic time-stepping   Contents
mitgcm-support@mitgcm.org
Copyright © 2006 Massachusetts Institute of Technology Last update 2011-01-09