|
|
|
|



Next: 1.3.2 Atmosphere
Up: 1.3 Continuous equations in
Previous: 1.3 Continuous equations in
Contents
Subsections
at fixed and moving 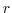 surfaces we set (see figure 1.15):
surfaces we set (see figure 1.15):
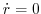 at at 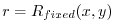 (ocean bottom, top of the atmosphere) (ocean bottom, top of the atmosphere) |
(1.7) |
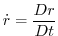 at at  (ocean surface,bottom of the atmosphere) (ocean surface,bottom of the atmosphere) |
(1.8) |
Here
where
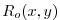 is the `
is the `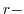 value' (height or pressure, depending on
whether we are in the atmosphere or ocean) of the `moving surface' in the
resting fluid and
value' (height or pressure, depending on
whether we are in the atmosphere or ocean) of the `moving surface' in the
resting fluid and  is the departure from
is the departure from
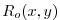 in the presence
of motion.
in the presence
of motion.
 |
(1.9) |
where
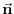 is the normal to a solid boundary.
is the normal to a solid boundary.



Next: 1.3.2 Atmosphere
Up: 1.3 Continuous equations in
Previous: 1.3 Continuous equations in
Contents
mitgcm-support@mitgcm.org
| Copyright © 2006
Massachusetts Institute of Technology |
Last update 2018-01-23 |
 |
|